Chapter 2. Seki Takakazu

With respect to angle α, assume

.

Then, we obtain

.

By repeating this, we obtain

.

And now, as

holds true due to

,

the Addition Theorem with respect to tan,

or

is derived.

J. Machin, an astronomer from London, discovered that

held true when

, and he calculated Pi to 100 decimal points in 1706 by using this relationship and

.

When we actually calculate

with an 8-digit calculator, we obtain a figure of 3.1415918.

Subsequently, relational expressions were discovered including the following:

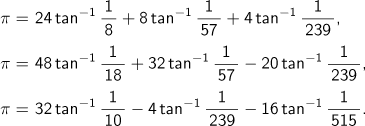
Using such expressions, calculations to evaluate Pi to still further decimal places were attempted.